A right triangle. The angle of interest is near the cartoon person and is labeled theta. The side of the triangle low down between the angle of interest and the right angle, the adjacent side, is labeled little d (distance). The side of the triangle opposite the angle of interest is labeled big D (physical size). The big D side of the triangle stretches across the full length of the cartoon galaxy.

The sizes of viewed objects can be given in terms of their angular size as seen by an observer rather than their physical size. As portrayed in the picture above, the distance to the object being observed d, the physical size of the object D, and the angular size of the object θ (in radians) all form a right triangle with the trigonometric relationship:

The equation reads, "Tangent of theta equals big D (physical size) over little d (distance)"

The Small Angle Approximation for trigonometry states that:

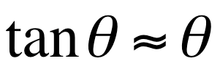
The equation reads, "Tangent of theta approximately equals theta".

The Small Angle Formula can be applied when θ is small (< 10°), or when d >> D (much greater - not just a couple times as large, but a few, 10, even 100+ times as large). The angular sizes of many objects in the sky are small and the Small Angle Approximation can be applied when studying them. If we take the Small Angle Approximation and substitute it into our first equation, that equation then becomes: 

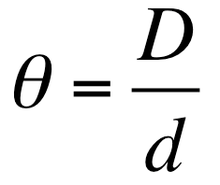
The equation reads, 'Theta equals big D (physical size) over little d (distance)'.

which is called the Small Angle Formula, where again θ must be an angular size in radians. 

θ is in units of radians, but we will sometimes have angular size measurements in units of degrees. Using the fact that 1 radian is 360/2π ≈ 57.3 degrees, we can rewrite the Small Angle Formula so that θ can be input as an angular size in degrees:

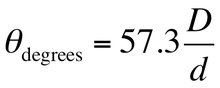
The equation reads, 'Theta in degrees equals 57.3 times big D (physical size) over little d (distance)'.

When dealing with astronomically distant objects, angular sizes are extremely small, and it is often more practical to use angular size measurements in units of arcseconds (") instead of degrees, where 1 arcsecond is 1/3600th of 1 degree.  So 1 radian is (3600 x 360)/2π ≈ 206265 arcseconds, and we can again rewrite the Small Angle Formula, now as:

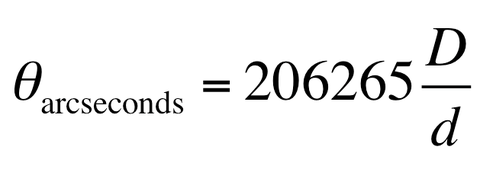
The equation reads, 'Theta in arcseconds equals 206265 times big D (physical size) over little d (distance)'.

Is is easy to measure θ, the angular size of astronomical objects, so we often use the Small Angle Formula to solve for other unknowns (either D or d). If we know the distance d to an object we are observing, we can then use it with the angular size θ and the Small Angle Formula to find the physical size D of that celestial object. Or if two objects are roughly the same distance d from the observer, we can use that with the angular size θ and the Small Angle Formula to find the distance between the two objects (where here D is the distance between the two objects). Additionally, some objects in space have a 'typical size', a physical diameter D that we know is a good rough approximation for the size. When we know the values of both θ and D, we can use them with the Small Angle Formula to find the distance d to the celestial object. 

Example Question

On August 27, 2003, Mars made the closest approach to Earth in recorded history due to a near synchronization of Earth being at aphelion (the furthest point out in its orbit around the Sun) and Mars being at perihelion (the closest point to the Sun in its orbit around the Sun). The distance between the two planets that day was a mere 55.8 million km (kilometers). Observations of Mars were quite easy to make that night, since the angular diameter of the planet was quite large at about 25.1" (arcseconds). Given the information here, find the diameter of the Red Planet in km. 

Solution

d = 55.8 million km = 55.8 x 106 km; θ = 25.1" 

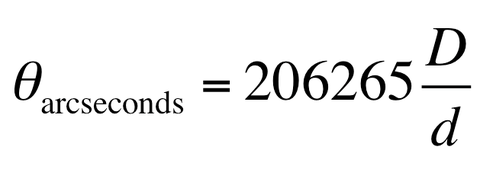
The equation reads, 'Theta in arcseconds equals 206265 times big D (physical size) over little d (distance)'.
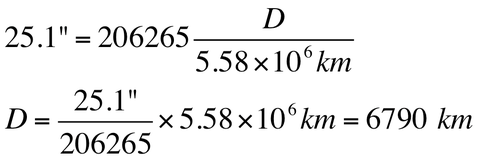
The worked out equations read, '25.1 arcseconds equals 206265 times big D (physical size) over 5.58 times 10 to the power of 6 kilometers', and 'Big D (physical size) equals 25.1 arcseconds over 206265 times 5.58 times 10 to the power of 6 kilometers equals 6790 kilometers'.

Mars' diameter D is about 6790 km, a little more than half the diameter of Earth.