Questions:
1. Explain what kind of observations you would use to measure the parallax of Alpha Centauri. It may be helpful to examine the image at the top of this page.
The Small Angle Formula takes on a special form if we force d and D into the units of parsecs, where
1 parsec ~ 206265 AU,
and rewriting θ = p in arcseconds, the small angle formula is then simply
,
where p is the parallax angle observed in arcseconds, and D is the actual distance measured in parsecs.
2. Find the distance to Alpha Centauri. Show your work and put your answer in parsecs and AU.
One of the limitations of determining distance using parallax is the resolving power of the telescope you are using to take the images. Telescopes with high resolution can resolve smaller objects or separations than telescopes with low resolution. Rigel, a telescope operated by the University of Iowa at the Iowa Robotic Observatory in Arizona, can resolve objects larger than about 3 arcseconds.
Since each telescope has a different resolution limit, images taken with different telescopes will have varying properties as well. One of these properties is how the pixel scale of the image relates to size. You are probably more familiar with relating pixels to inches, or cm, which is commonly used software that processes photos. In astronomy, relating pixels directly to a distance is difficult, since we rarely know how far away astronomical objects are. Instead, the pixel scale is related to angular size. The image scale for Rigel, which relates the pixel size in the image to an angular size, is 0.73 arc seconds per pixel.
3. Using the Rigel image scale, determine the resolution limit of Rigel in pixels. Show your work.
Now that you know the size of the small object in pixels that Rigel can resolve, you can determine if you can use Rigel to observe the apparent shift in position of Alpha Centauri using the observing scheme you detailed in question 1.
4. Using the Rigel image scale and the known parallax angle of Alpha Centauri, determine how pixels Alpha Centauri would shift between the two images taken 6 months apart with Rigel . Compare your answer to the resolution limit of Rigel. How would your answer affect the calculation of the distance to Alpha Centauri? Show your work.
5. Hubble Space Telescope has a resolution of 0.1 arcseconds. If you could use Hubble to find the parallax of a star instead of Rigel, what is the distance of the farthest star you could measure? Is such a star in our Galaxy still? (Hint, our Galaxy is 100,000 lightyears wide and we are ~27,000 lightyears from the center of our Galaxy.) Show your work.
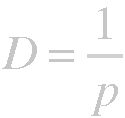
Stellar Parallax
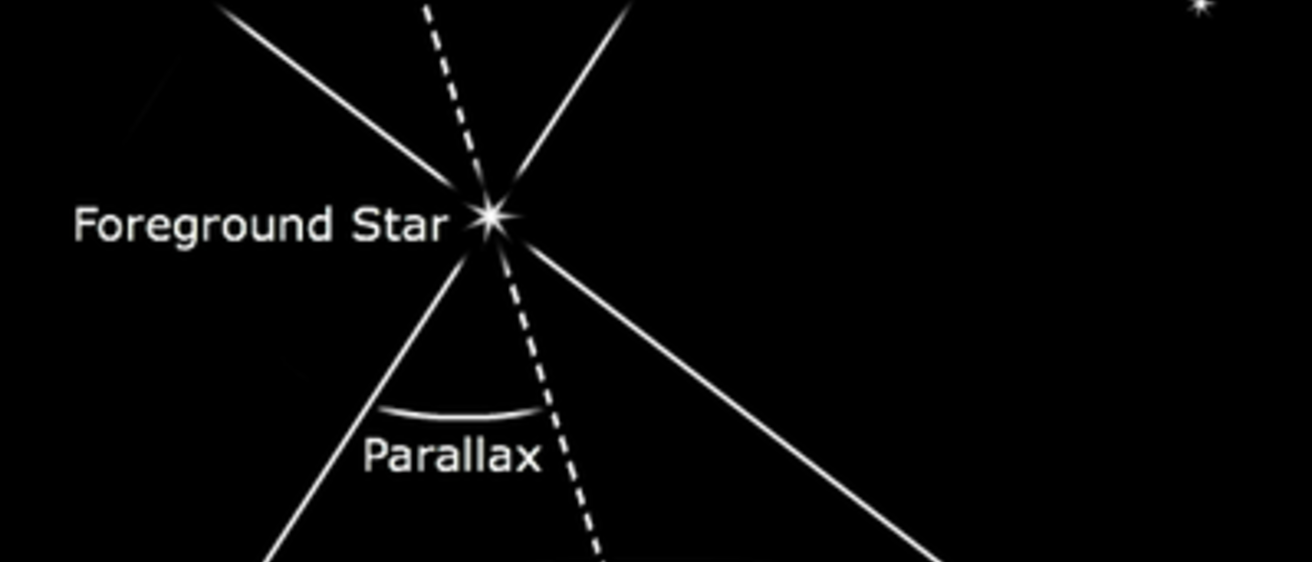
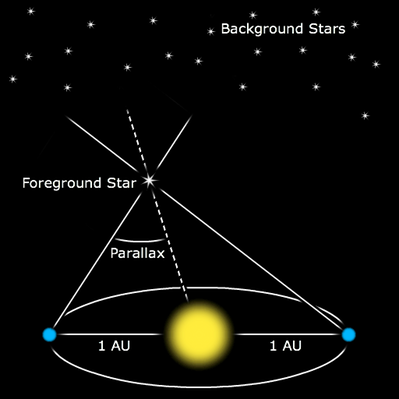
Parallax is the observed apparent change in the position of an object resulting from a change in the position of the observer. Specifically, in the case of astronomy it refers to the apparent displacement of a nearby star as seen from an observer on Earth.
The apparent displacement of a nearby star is an observed angle, so we can use the Small Angle Formula to find the distance to the star.
Since we can measure the angular shift in apparent position over a six month time period (see the image above), we set d equal to 1 AU and will solve for D, the distance to the star.
Read the following paragraph concerning the star Alpha Centauri and answer the questions below.
Alpha Centauri (α Cen) is the nearest star system to the Sun. It is the third brightest star in the night sky, after Sirius and Canopus, but lies so far south that it is visible only from latitudes below 25° N. Its two bright components, A and B, consist of a yellow G star, similar to the Sun but about 200 million years older, and an orange K star of the same age.
These two stars are moving around each other every 79.9 years in a highly elongated orbit (eccentricity 0.519) with a mean separation of 23.7 AU, which is a bit more than the distance between Uranus and the Sun. The angular separation between the two stars is ~17.7 arcseconds. Biannual observations of the binary star system shows an apparent change in position of ~ 0.75 arcseconds. No planets have been found in the system to date.